The lights are dim, as our hero contemplates his cards in a dingy room filled with cigarette smoke. He looks mournfully at the large stack of chips in front of him. Suddenly, his eyes widen as a thought rips its way through his brain. The protagonist pushes all his chips into the middle of the table.
Many fictional depictions of poker end in a scene like this, in which the entire game hinges on one single moment. If the opponent has the cards, hours of flawless play are rendered irrelevant. But if they do not, all is forgiven, and the protagonist can snatch victory from the jaws of defeat. Magic often has moments like this as well. When the combo deck goes for it into an ominous two blue mana, when the aggro deck makes an all-out attack that trades multiple creatures for exactly lethal, or when the Affinity deck sacrifices everything to Arcbound Ravager: these are times when the game is decided that turn, by that decision. This article is about when to shove all your chips into the middle and when to back off and fight another day.
Assessing the Situation: The Three Questions
The first question to ask yourself before you go for the throat is: “How bad will this be for me if it goes wrong?” Sometimes, the answer is “not that bad.” For instance, let’s say you control five 1/1s and your opponent has a 2/2, with 4 life to your 20. If you attack with everything and they have an instant-speed removal spell, you still deal them three points of damage and have lethal through a blocker again next turn. As a result, you should almost certainly go for it here. But let’s say instead they have four 2/2 creatures but are at one life against your five 1/1s. Now, if you go for the win, and they have something, you stand almost no chance of getting back into the game.
That means it’s time for the second question: How likely is it that they have the answer? To assess this question, you need to consider what exactly they would need to have and then how probable it is for them to have that card or combination of cards. Considering what beats you is mostly a math problem. Let’s say your Modern Jund opponent has just a Blackcleave Cliffs untapped on Turn 5 and needs to have a removal spell to survive. The typical Jund list has six copies of Lightning Bolt / Fatal Push in some mixture. Thus, they are around 40% to have it, assuming they have not cast any copies of either so far.
I don’t really have amazing advice for estimating math quickly in your head, but if you are playing a deck that often ends up in these spots, I recommend messing around with a hypergeometric calculator to get a sense of the more common scenarios. As a rule of thumb, I rarely play around multiples of a specific card but I sometimes play around multiples of a type of card.
The third question is, “If my opponent had drawn a copy of their answer earlier in this game, would they have used it?” If the answer is yes, you only need to count the draw steps since they would have cast it. Let’s say you’re playing a Standard Azorius Control mirror and three turns ago you cast a Teferi, Time Raveler into your opponent’s three open mana. It resolved but your opponent ultimately removed it. Now, you are trying to assess whether to tap out for a game-winning threat into their open mana. If they had any counterspell the turn you went for Teferi, they certainly would have cast it rather than have it stranded in their hand. Since they didn’t, you can conclude that they had no counterspells at that time. That means they had only the three intervening draw steps to find one.
Although full calculation depends on a bunch of details like how many counterspells are in their deck and the number of cards they’ve drawn, the odds of having drawn it in a handful of turns are almost always under 50%. That means it is generally correct to tap out in this situation. On the other hand, I generally assume that if they have had no opportunity yet to cast a problematic effect, and they run four or more copies, they have it.
Desperation
Let’s imagine that you ‘re dead on your opponent’s next turn in a manner you cannot stop at all. In that situation, you would obviously go all-in even if it’s extremely unlikely to work since some chance of victory is better than none. By similar logic, the more behind you are in a game, the more you should be willing to go all-in (and vice versa). To be precise, if you know that going for the throat is about 40% to work, but you are only 30% to win a long game, you should go for it even though you will most likely lose on the spot. By the same token, if you are 65% to win the game if it goes long, and trying to win now is 60% to work, you should play for the long-game despite being better-than-even to win right now.
Obviously, it’s unrealistic to know your exact odds of winning any given game but you can try to get a sense by thinking about how behind you are and how much needs to go right to win in the long run. It’s worth getting good at this skill, as it will help you in many areas of Magic.
Is It Getting Better?
Sometimes you find yourself playing a deck that basically only wins by going all-in (think Storm, not Splinter Twin). If you’re playing a deck like that, you must ask yourself whether your odds get better or worse with each turn you wait. If your Storm deck has four discard spells and your opponent’s control deck has ten counterspells and plenty of lands on the battlefield, each draw step is going to make it harder for you to win, on average. Thus, you should almost certainly go all-in as soon as you can, even if it isn’t that likely to work.
Unbeatable Cards
A common mistake I see mid-level players make is playing around too much. To paraphrase Craig Wescoe, make sure you can beat what’s on the battlefield before playing around cards that may be in your opponent’s hand (if you’re at two life, you don’t need to worry about Shock, for example). In the Jund example I discussed earlier, you don’t need to worry about how likely you are to win the long game in general; you need to worry about how likely you are to win the long-game if they have a one-mana removal spell. Even if you don’t go for it, they still get to cast the card on your end step. If that’s devastating anyway, you might as well go for the win.
A Practical Example
The following screenshot comes from the last round of the Modern Challenge last weekend. I was playing Mono-Red Prowess against Heliod Company. In Game 3, I reached the game state below. It’s a little small so just to be clear:
I cast a Crash Through earlier this turn so the Monastery Swiftspears are currently 2/3s with trample.
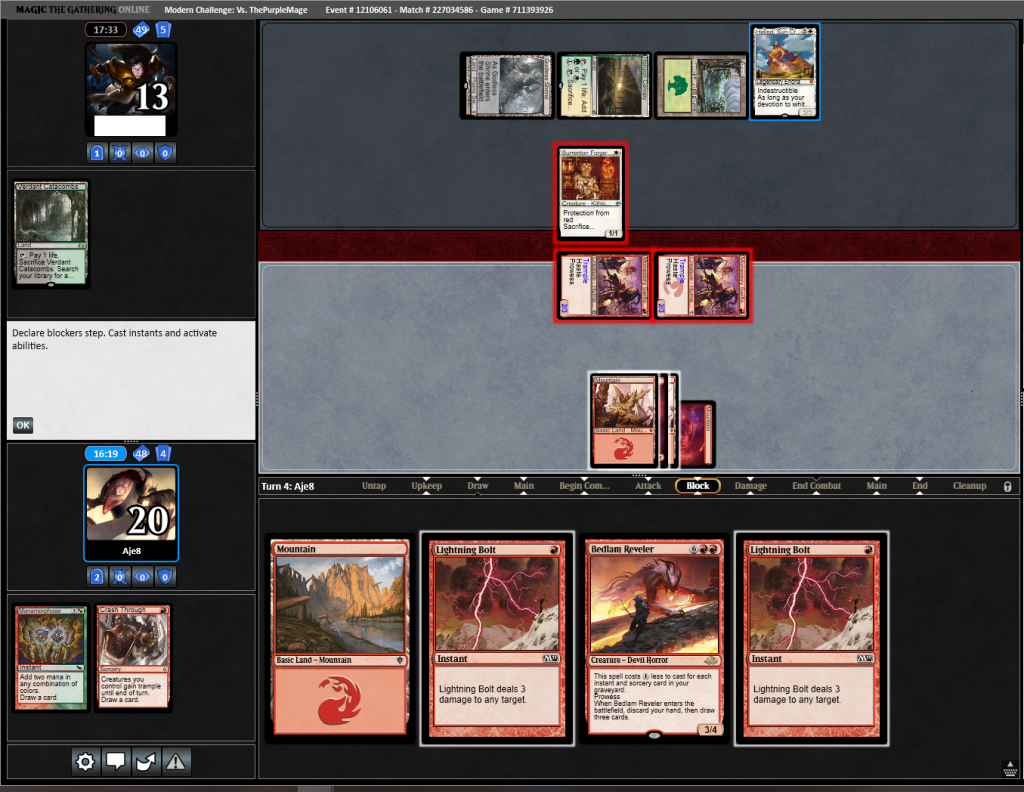
There are only two realistic options here: cast both Lightning Bolts targeting our opponent’s head or go straight to damage.
If I cast both Bolts, both Monastery Swiftspears will be 4/5s. Because they have trample, my opponent is going to take 4 + 4 + 3 + 3 – 1 = 13 damage. Thus, they will be forced to sacrifice Burrenton Forge-Tender to survive, and go to two life to boot. Next turn, I can cast Bedlam Reveler and have three cards to kill them. That is close to a guaranteed win.
But if I take that line and my opponent has Spike Feeder in hand, I lose the game on the spot. My deck is incapable of beating infinite life and my opponent already has Heliod, Sun-Crowned on the battlefield. Holding back both Bolts prevents Spike Feeder from killing me.
At this point I asked myself what the odds were that my opponent was holding Spike Feeder. I reasoned that because my opponent kept seven cards this game, it was unlikely that all they could muster was a Burrenton Forge-Tender, no two-drop on Turn 2, and a Heliod on Turn 3. If they didn’t have the Spike Feeder, why was Heliod their best play last turn? We know their hand cannot contain any cards that cost one or two mana and we know that, if they don’t have Spike Feeder, their hand has no better three-drops either. Are their five cards really all four-drops, lands, and additional copies of Heliod? That seems incredibly unlikely. I estimate that there’s at most a 5% chance that they don’t have the Spike Feeder here. Those odds mostly account for my opponent just inexplicably keeping a bad hand.
But there is one final important factor. What does the game look like if I do not cast either Bolt? My opponent will cast Spike Feeder on their turn. Then, crucially, they can just not activate its ability. If they don’t activate it, I cannot act. If I Bolt Spike Feeder, they can remove a counter in response, counter my second Bolt with Forge-Tender, and gain infinite life.
Thus, my only choice is to untap and waste my mana. If I topdeck a third removal spell, I can spend all three of them to remove Spike Feeder and Forge-Tender. Then I can attack my opponent (who is now at 13 from Spike Feeder) for eight damage and with Reveler on the horizon, I’m decently ahead. But if I do not topdeck a third removal spell, I am very unlikely to win. After all, if my opponent casts a second Forge-Tender, a Ranger-Captain of Eos, or any card that gains life next turn, I lose on the spot. Since their hand is likely all three- and four-drops, it’s very likely my opponent has such a card.
This means the line of going to damage has odds of winning equal to my odds of topdecking a removal spell and then converting the resulting decent position. I couldn’t do the exact math at the table, but I knew I had fourteen removal spells remaining in my deck and seven cheap cantrips. According to the hypergeometric calculator, that means I am roughly 30% to keep battling with the cast-nothing line and about 60% to win from there assuming that he has Spike Feeder.
60% of 30% is 18%, which is a lot more than my estimate of 5%, so I held onto my cards and lived to fight another day.
I hoped this article helps at least one person go all-in for the win. Getting these decisions right is one of the most useful skills a player can learn and will help you steal games you’re losing and hold tight to games you’re winning. As for the match I mentioned above, here’s what happened:









